Heat Transfer

 

Your cousin takes some hot cookies out of the oven and puts them on a rack to cool down. He notices that while the cookies are cooling down the temperature of the kitchen does not change much at all. He wonders how it is possible for the temperature of the cookies to change so much while the temperature of the kitchen does not change. Explain how this is possible. (Use diagrams, graphs, words and videos)

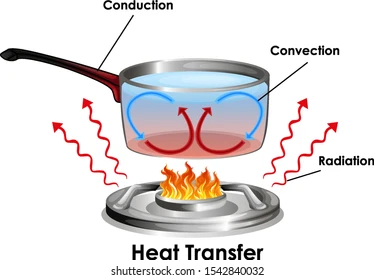
Conduction : is the transfer of thermal energy between two objects from the object which has more energy to the object which has less energy through direct contact.

Convection :the transfer of thermal energy through the movement of liquid or gas particles . Like  when the particles of the liquid gain heat so they become faster and lighter ,go up and the cooler particles go down and so on  in a circulation.

Radiation : is the transfer of energy through thermal emission.

For our experiment here we have two explanations :...

Conduction the transfer of heat from the cookies to the rack through direct contact.

Then convection ,  when the heat energy transferred from the hot cookies to the air particles that surrounded the kitchen, however the kitchen temperature didn’t change 

Because  the difference in the heat energy was not enough to raise the temperature of the kitchen

https://youtu.be/e9H2DDKgnr4?si=XQ_JXk7N3MzUSHPH